Triangles and TrigonometryPythagoras’ Theorem

We have now reached an important point in geometry – being able to state and understand one of the most famous theorems in all of mathematics: Pythagoras’ Theorem. It is named after the ancient Greek mathematician Pythagoras of Samos.

Pythagoras’ Theorem
In any right-angled triangle, the square of the length of the hypotenuse (the side that lies opposite the right angle) is equal to the sum of the squares of the other two sides. In other words,

a2 + b2 = c2

The converse is also true: if the three sides in a triangle satisfy a2+b2=c2, then it must be .

Right angles are everywhere, and that’s why Pythagoras’ Theorem is so useful.

Here you can see a 6m long ladder leaning on a wall. The bottom of the ladder is 1m away from the wall. How far does it reach up the wall?

Notice that there is a right-angled triangle formed by the ladder, the wall and the ground. Using Pythagoras’ theorem, we get

h2+12	=62
⇒h2	=
⇒h	=35=5.92m

Whenever you’ve got a right-angled triangle and know two of its sides, Pythagoras can help you find the third one.

Proving Pythagoras’ Theorem

Pythagoras’ theorem was known to ancient Babylonians, Mesopotamians, Indians and Chinese – but Pythagoras may have been the first to find a formal, mathematical proof.

There are actually many different ways to prove Pythagoras’ theorem. Here you can see three different examples that each use a different strategy:

Rearrangement

Have a look at the figure on the right. The square has side length a+b, and contains four right-angled triangles, as well as a smaller square of area .

Now let’s rearrange the triangles in the square. The result still contains the four right-angles triangles, as well as two squares of size .

Comparing the area of the red area and the rearrangement, we see that

a2+b2=c2.

This is the original proof that Pythagoras came up with.

c²

a²

b²

Algebra

Here we have the same figure as before, but this time we’ll use algebra rather than rearrangement to prove Pythagoras’ theorem.

The large square has side length a+b and area .

It consists of four triangles, each with an area of , and one square of area .

If we combine all of that information, we have

a+b2	=4×12ab+c2
a2+2ab+b2	=2ab+c2
a2+b2	=c2

And, once again, we get Pythagoras’ theorem.

Similar Triangles

Here you can see another right-angled triangle. If we draw one of the altitudes, it splits the triangle into two smaller triangle. It also divides the hypotenuse c into two smaller parts which we’ll call x and y. Continue

Let’s separate out the two smaller triangles, so that it’s clearer to see how they are related… Continue

Both smaller triangles share one angle with the original triangle. They also all have one right angle. By the AA condition, all three triangles must be .

Now we can use the equations we already know about similar polygons:

xa=ac
x=a2c

yb=bc
y=b2c

But remember that c=x+y. Therefore

c=a2c+b2c

c2=a2+b2

Once more, we’ve proven Pythagoras’ theorem!

Much about Pythagoras’ life is unknown, and no original copies of his work have survived. He founded a religious cult, the Pythagoreans, that practiced a kind of “number worship”. They believed that all numbers have their own character, and followed a variety of other bizarre customs.

The Pythagoreans are credited with many mathematical discoveries, including finding the first irrational number, 2. Irrational numbers cannot be expressed as a simple fraction – a concept the Pythagoreans found deeply troubling and (unsuccessfully) tried to cover up!

“Pythagoreans celebrate sunrise” by Fyodor Bronnikov

Calculating Distances

One of the most important application of Pythagoras’ Theorem is for calculating distances.

On the right you can see two points in a coordinate system. We could measure their distance using a ruler, but that is not particularly accurate. Instead, let’s try using Pythagoras.

We can easily count the horizontal distance along the x-axis, and the vertical distance along the y-axis. If we draw those two lines, we get a right-angled triangle.

Using Pythagoras,

d2	=${b.x-a.x}2+${b.y-a.y}2
d2	=${(b.x-a.x)2 + (b.y-a.y)2}
d	=${(b.x-a.x)2 + (b.y-a.y)2}=${round(distance(a,b),4)}

This method works for any two points:

The Distance Formula
If you are given two points with coordinates (x1,y1) and (x2,y2), the distance between them is

d2=x2−x12+y2−y12

d=x2−x12+y2−y12

Pythagorean Triples

As you moved the vertices of the triangle in the previous step, you might have noticed that in most cases, the length of the hypothenuse d ended up being a . However there are a few examples of right-angled triangles where the lengths of all three sides happens to be whole numbers.

One famous example is the 3-4-5 triangle. Since 32+42=52, any triangle with sides of length 3, 4 and 5 must be right-angled.

The ancient Egyptians didn’t know about Pythagoras’ theorem, but they did know about the 3-4-5 triangle. When building the pyramids, they used knotted ropes of lengths 3, 4 and 5 to measure perfect right angles.

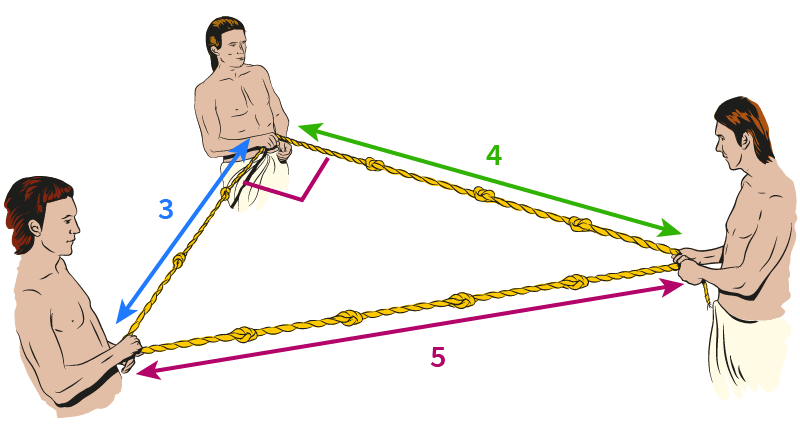
Three integers like this are called Pythagorean Triples. (3, 4, 5) is one example of a Pythagorean triple. If we multiply every number by 2, we get another Pythagorean triple: (6, 8, ).

We can think of these triples as grid points in a coordinate systems. For a valid Pythagorean triples, the distance from the origin to the grid point has to be a whole number. Using the coordinate system below, can you find any other Pythagorean triples?

${a.x}

${a.y}

${round(a.length,2)}

Do you notice any pattern in the distribution of these points?

Verander de taal

Log in bij Mathigon

Delen

Voortgang resetten

Woordenlijst